Tag Archives: binary tree
C# || How To Determine If A Binary Tree Is Even Odd Tree Using C#

The following is a module with functions which demonstrates how to determine if a binary tree is an even odd tree using C#.
1. Is Even Odd Tree – Problem Statement
A binary tree is named Even-Odd if it meets the following conditions:
- The root of the binary tree is at level index 0, its children are at level index 1, their children are at level index 2, etc.
- For every even-indexed level, all nodes at the level have odd integer values in strictly increasing order (from left to right).
- For every odd-indexed level, all nodes at the level have even integer values in strictly decreasing order (from left to right).
Given the root of a binary tree, return true if the binary tree is Even-Odd, otherwise return false.
Example 1:
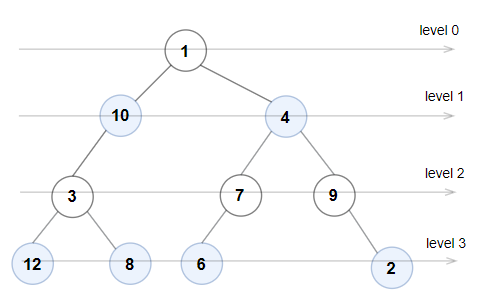
Input: root = [1,10,4,3,null,7,9,12,8,6,null,null,2]
Output: true
Explanation: The node values on each level are:
Level 0: [1]
Level 1: [10,4]
Level 2: [3,7,9]
Level 3: [12,8,6,2]
Since levels 0 and 2 are all odd and increasing and levels 1 and 3 are all even and decreasing, the tree is Even-Odd.
Example 2:
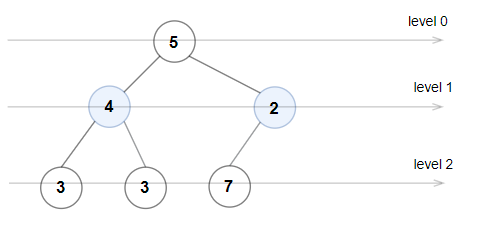
Input: root = [5,4,2,3,3,7]
Output: false
Explanation: The node values on each level are:
Level 0: [5]
Level 1: [4,2]
Level 2: [3,3,7]
Node values in level 2 must be in strictly increasing order, so the tree is not Even-Odd.
Example 3:
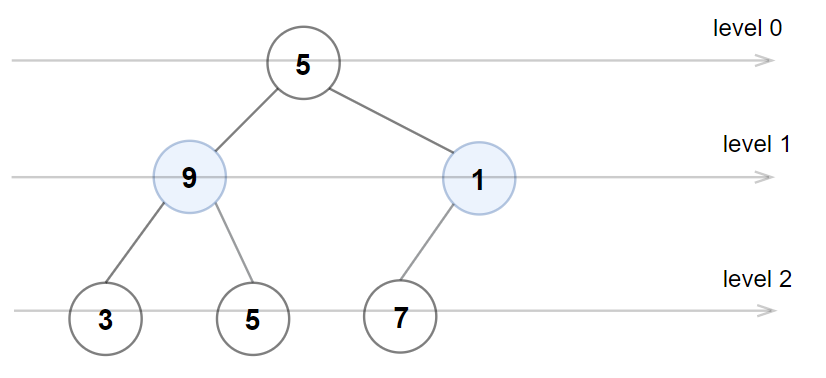
Input: root = [5,9,1,3,5,7]
Output: false
Explanation: Node values in the level 1 should be even integers.
2. Is Even Odd Tree – Solution
The following is a solution which demonstrates how to determine if a binary tree is an even odd tree.
This solution uses breadth-first search.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 |
// ============================================================================ // Author: Kenneth Perkins // Date: Mar 1, 2024 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to determine an even odd tree // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public bool IsEvenOddTree(TreeNode root) { var queue = new Queue<TreeNode>(); queue.Enqueue(root); var isEvenLevel = true; while (queue.Count > 0) { int previousValue = isEvenLevel ? int.MinValue : int.MaxValue; for (var size = queue.Count - 1; size >= 0; --size) { var currentNode = queue.Dequeue(); if (isEvenLevel) { if (IsEven(currentNode.val) || previousValue >= currentNode.val) { return false; } } else { if (!IsEven(currentNode.val) || previousValue <= currentNode.val) { return false; } } if (currentNode.left != null) { queue.Enqueue(currentNode.left); } if (currentNode.right != null) { queue.Enqueue(currentNode.right); } previousValue = currentNode.val; } isEvenLevel = !isEvenLevel; } return true; } private bool IsEven(int value) { return value % 2 == 0; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
true
false
false
C# || Parallel Courses III – How To Find Minimum Number Months To Complete All Courses Using C#

The following is a module with functions which demonstrates how to find the minimum number of months needed to complete all courses using C#.
1. Minimum Time – Problem Statement
You are given an integer n, which indicates that there are n courses labeled from 1 to n. You are also given a 2D integer array relations where relations[j] = [prevCoursej, nextCoursej] denotes that course prevCoursej has to be completed before course nextCoursej (prerequisite relationship). Furthermore, you are given a 0-indexed integer array time where time[i] denotes how many months it takes to complete the (i+1)th course.
You must find the minimum number of months needed to complete all the courses following these rules:
- You may start taking a course at any time if the prerequisites are met.
- Any number of courses can be taken at the same time.
Return the minimum number of months needed to complete all the courses.
Note: The test cases are generated such that it is possible to complete every course (i.e., the graph is a directed acyclic graph).
Example 1:

Input: n = 3, relations = [[1,3],[2,3]], time = [3,2,5]
Output: 8
Explanation: The figure above represents the given graph and the time required to complete each course.
We start course 1 and course 2 simultaneously at month 0.
Course 1 takes 3 months and course 2 takes 2 months to complete respectively.
Thus, the earliest time we can start course 3 is at month 3, and the total time required is 3 + 5 = 8 months.
Example 2:
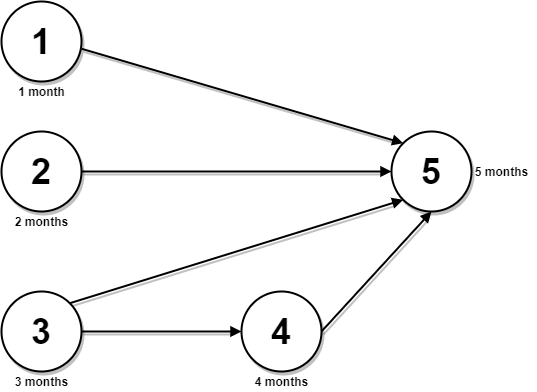
Input: n = 5, relations = [[1,5],[2,5],[3,5],[3,4],[4,5]], time = [1,2,3,4,5]
Output: 12
Explanation: The figure above represents the given graph and the time required to complete each course.
You can start courses 1, 2, and 3 at month 0.
You can complete them after 1, 2, and 3 months respectively.
Course 4 can be taken only after course 3 is completed, i.e., after 3 months. It is completed after 3 + 4 = 7 months.
Course 5 can be taken only after courses 1, 2, 3, and 4 have been completed, i.e., after max(1,2,3,7) = 7 months.
Thus, the minimum time needed to complete all the courses is 7 + 5 = 12 months.
2. Minimum Time – Solution
The following is a solution which demonstrates how to find the minimum number of months needed to complete all courses.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 1, 2023 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to find the minimum months complete courses // ============================================================================ public class Solution { public int MinimumTime(int n, int[][] relations, int[] time) { var graph = new Dictionary<int, List<int>>(); for (int i = 0; i < n; i++) { graph[i] = new List<int>(); } int[] indegree = new int[n]; foreach (int[] edge in relations) { int x = edge[0] - 1; int y = edge[1] - 1; graph[x].Add(y); indegree[y]++; } var queue = new Queue<int>(); int[] maxTime = new int[n]; for (int node = 0; node < n; node++) { if (indegree[node] == 0) { queue.Enqueue(node); maxTime[node] = time[node]; } } while (queue.Count > 0) { int node = queue.Dequeue(); foreach (int neighbor in graph[node]) { maxTime[neighbor] = Math.Max(maxTime[neighbor], maxTime[node] + time[neighbor]); indegree[neighbor]--; if (indegree[neighbor] == 0) { queue.Enqueue(neighbor); } } } int ans = 0; for (int node = 0; node < n; node++) { ans = Math.Max(ans, maxTime[node]); } return ans; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
8
12
C# || How To Find Largest Value In Each Binary Tree Row Using C#

The following is a module with functions which demonstrates how to find the largest value in each binary tree row using C#.
1. Largest Values – Problem Statement
Given the root of a binary tree, return an array of the largest value in each row of the tree (0-indexed).
Example 1:
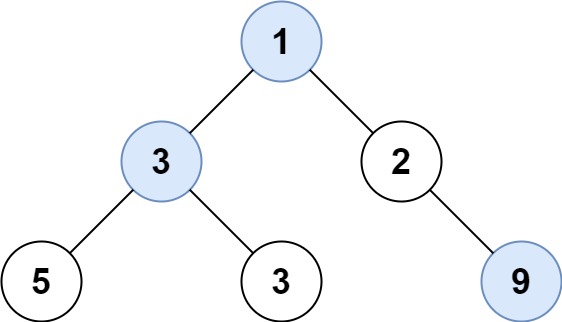
Input: root = [1,3,2,5,3,null,9]
Output: [1,3,9]
Example 2:
Input: root = [1,2,3]
Output: [1,3]
2. Largest Values – Solution
The following is a solution which demonstrates how to find the largest value in each binary tree row.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 |
// ============================================================================ // Author: Kenneth Perkins // Date: Oct 28, 2023 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to find the largest value binary tree row // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public IList<int> LargestValues(TreeNode root) { if (root == null) { return new List<int>(); } var ans = new List<int>(); var queue = new Queue<TreeNode>(); queue.Enqueue(root); while (queue.Count > 0) { int currMax = int.MinValue; for (int i = queue.Count -1; i >= 0; --i) { var node = queue.Dequeue(); currMax = Math.Max(currMax, node.val); if (node.left != null) { queue.Enqueue(node.left); } if (node.right != null) { queue.Enqueue(node.right); } } ans.Add(currMax); } return ans; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[1,3,9]
[1,3]
C# || How To Find Maximum Difference Between Node and Ancestor In Binary Tree Using C#

The following is a module with functions which demonstrates how to find the maximum difference between node and ancestor in binary tree using C#.
1. Max Ancestor Diff – Problem Statement
Given the root of a binary tree, find the maximum value v for which there exist different nodes a and b where v = |a.val – b.val| and a is an ancestor of b.
A node a is an ancestor of b if either: any child of a is equal to b or any child of a is an ancestor of b.
Example 1:
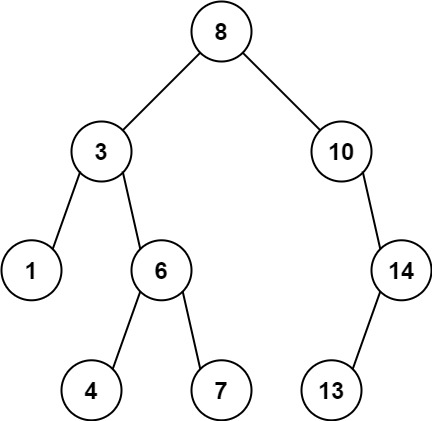
Input: root = [8,3,10,1,6,null,14,null,null,4,7,13]
Output: 7
Explanation: We have various ancestor-node differences, some of which are given below :
|8 - 3| = 5
|3 - 7| = 4
|8 - 1| = 7
|10 - 13| = 3
Among all possible differences, the maximum value of 7 is obtained by |8 - 1| = 7.
Example 2:
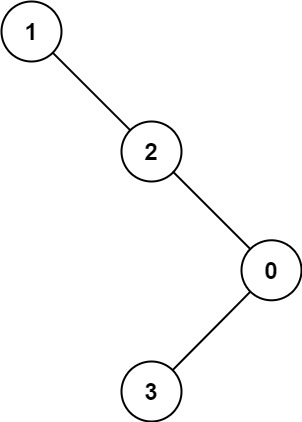
Input: root = [1,null,2,null,0,3]
Output: 3
2. Max Ancestor Diff – Solution
The following is a solution which demonstrates how to find the maximum difference between node and ancestor in binary tree.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 |
// ============================================================================ // Author: Kenneth Perkins // Date: Feb 14, 2023 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to find maximum difference node and ancestor // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int MaxAncestorDiff(TreeNode root) { if (root == null) { return 0; } return Traverse(root, root.val, root.val); } public int Traverse(TreeNode node, int curMax, int curMin) { // if encounter leaves, return the max-min along the path if (node == null) { return curMax - curMin; } // else, update max and min // and return the max of left and right subtrees curMax = Math.Max(curMax, node.val); curMin = Math.Min(curMin, node.val); int left = Traverse(node.left, curMax, curMin); int right = Traverse(node.right, curMax, curMin); return Math.Max(left, right); } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
7
3
C# || Two Sum IV – How To Get Two Numbers In Binary Search Tree Equal To Target Value Using C#

The following is a module with functions which demonstrates how to get two numbers in a binary search tree equal to target value using C#.
1. Find Target – Problem Statement
Given the root of a Binary Search Tree and a target number k, return true if there exist two elements in the BST such that their sum is equal to the given target.
Example 1:

Input: root = [5,3,6,2,4,null,7], k = 9
Output: true
Example 2:

Input: root = [5,3,6,2,4,null,7], k = 28
Output: false
2. Find Target – Solution
The following are two solutions which demonstrates how to get two numbers in a binary search tree equal to target value.
Both solutions use a set to keep track of the items already seen.
Each time a new node is encountered, we subtract the target value from the current node value. If the difference amount from subtracting the two numbers exists in the set, a 2 sum combination exists in the tree
1. Recursive
The following solution uses Depth First Search when looking for the target value.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 |
// ============================================================================ // Author: Kenneth Perkins // Date: Oct 9, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get two numbers equal to target value // ============================================================================ public class Solution { public bool FindTarget(TreeNode root, int k) { return Traverse(root, k, new HashSet<int>()); } private bool Traverse(TreeNode node, int k, HashSet<int> seen) { if (node == null) { return false; } // Get remaining value var remaining = k - node.val; // Check if remaining value has been seen if (seen.Contains(remaining)) { return true; } // Add current node value to items seen seen.Add(node.val); // Keep traversing left and right looking for 2 sum return Traverse(node.left, k, seen) || Traverse(node.right, k, seen); } }// http://programmingnotes.org/ |
2. Iterative
The following solution uses Breadth First Search when looking for the target value.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 |
// ============================================================================ // Author: Kenneth Perkins // Date: Oct 9, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get two numbers equal to target value // ============================================================================ public class Solution { public bool FindTarget(TreeNode root, int k) { if (root == null) { return false; } // Declare stack var stack = new Stack<TreeNode>(); // Keep track of values seen var seen = new HashSet<int>(); // Add root to stack stack.Push(root); // Loop through items on the stack while (stack.Count > 0) { // Get current node var node = stack.Pop(); // Get remaining value var remaining = k - node.val; // Check if remaining value has been seen if (seen.Contains(remaining)) { return true; } else { // Keep traversing left and right looking for 2 sum if (node.left != null) { stack.Push(node.left); } if (node.right != null) { stack.Push(node.right); } } // Add current node value to items seen seen.Add(node.val); } return false; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
true
false
C# || How To Traverse N-ary Tree Level Order Using C#

The following is a module with functions which demonstrates how to traverse a N-ary Tree level order using C#.
1. Level Order – Problem Statement
Given an n-ary tree, return the level order traversal of its nodes’ values.
Nary-Tree input serialization is represented in their level order traversal, each group of children is separated by the null value (See examples).
Example 1:
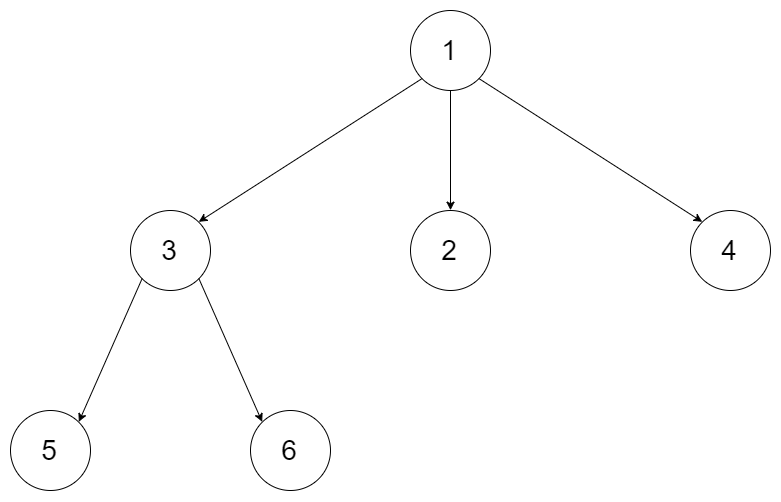
Input: root = [1,null,3,2,4,null,5,6]
Output: [[1],[3,2,4],[5,6]]
Example 2:
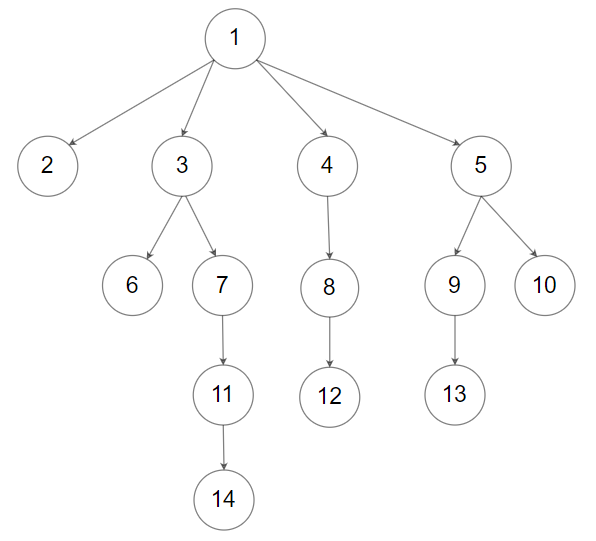
Input: root = [1,null,2,3,4,5,null,null,6,7,null,8,null,9,10,null,null,11,null,12,null,13,null,null,14]
Output: [[1],[2,3,4,5],[6,7,8,9,10],[11,12,13],[14]]
2. Level Order – Solution
The following is a solution which demonstrates how to traverse a N-ary Tree level order.
This solution uses Breadth First Search to explore items at each level.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 |
// ============================================================================ // Author: Kenneth Perkins // Date: Sep 5, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to traverse a N-ary Tree Level Order // ============================================================================ /* // Definition for a Node. public class Node { public int val; public IList<Node> children; public Node() {} public Node(int _val) { val = _val; } public Node(int _val, IList<Node> _children) { val = _val; children = _children; } } */ public class Solution { public IList<IList<int>> LevelOrder(Node root) { var result = new List<IList<int>>(); if (root == null) { return result; } var queue = new Queue<Node>(); queue.Enqueue(root); while (queue.Count > 0) { var currentLevel = new List<int>(); for (int count = queue.Count - 1; count >= 0 ; --count) { var currentNode = queue.Dequeue(); currentLevel.Add(currentNode.val); foreach (var child in currentNode.children) { queue.Enqueue(child); } } result.Add(currentLevel); } return result; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[[1],[3,2,4],[5,6]]
[[1],[2,3,4,5],[6,7,8,9,10],[11,12,13],[14]]
C# || How To Validate A Binary Search Tree Using C#

The following is a module with functions which demonstrates how to validate a binary search tree using C#.
1. Is Valid BST – Problem Statement
Given the root of a binary tree, determine if it is a valid binary search tree (BST).
A valid BST is defined as follows:
- The left subtree of a node contains only nodes with keys less than the node’s key.
- The right subtree of a node contains only nodes with keys greater than the node’s key.
- Both the left and right subtrees must also be binary search trees.
Example 1:

Input: root = [2,1,3]
Output: true
Example 2:

Input: root = [5,1,4,null,null,3,6]
Output: false
Explanation: The root node's value is 5 but its right child's value is 4.
2. Is Valid BST – Solution
The following is a solution which demonstrates how to validate a binary search tree.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 |
// ============================================================================ // Author: Kenneth Perkins // Date: Aug 12, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to validate a BST // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public bool IsValidBST(TreeNode root) { return Traverse(root, null, null); } private bool Traverse(TreeNode node, TreeNode min, TreeNode max) { if (node == null) { return true; } if (max != null && node.val >= max.val) { return false; } if (min != null && node.val <= min.val) { return false; } return Traverse(node.left, min, node) && Traverse(node.right, node, max); } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
true
false
C# || Binary Tree Right Side View – How To Get Nodes Ordered Top To Bottom C#

The following is a module with functions which demonstrates how to get nodes in a binary tree ordered from top to bottom using C#.
1. Right Side View – Problem Statement
Given the root of a binary tree, imagine yourself standing on the right side of it, return the values of the nodes you can see ordered from top to bottom.
Example 1:

Input: root = [1,2,3,null,5,null,4]
Output: [1,3,4]
Example 2:
Input: root = [1,null,3]
Output: [1,3]
Example 3:
Input: root = []
Output: []
2. Right Side View – Solution
The following is a solution which demonstrates how to get right side nodes ordered from top to bottom.
This solution uses Depth First Search level order traversal to explore items at each level, and then adds the last node on every layer.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 |
// ============================================================================ // Author: Kenneth Perkins // Date: Jul 10, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get nodes ordered from top to bottom // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public IList<int> RightSideView(TreeNode root) { var result = new List<int>(); Traverse(root, 0, result); return result; } public void Traverse(TreeNode node, int currentDepth, List<int> result) { if (node == null) { return; } if (currentDepth == result.Count) { result.Add(node.val); } Traverse(node.right, currentDepth + 1, result); Traverse(node.left, currentDepth + 1, result); } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[1,3,4]
[1,3]
[]
C# || How To Get Total Sum Root To Leaf Binary Numbers In Binary Tree Using C#

The following is a module with functions which demonstrates how to get the total sum root to leaf binary numbers in a binary tree using C#.
1. Sum Root To Leaf – Problem Statement
You are given the root of a binary tree where each node has a value 0 or 1. Each root-to-leaf path represents a binary number starting with the most significant bit.
- For example, if the path is 0 -> 1 -> 1 -> 0 -> 1, then this could represent 01101 in binary, which is 13.
For all leaves in the tree, consider the numbers represented by the path from the root to that leaf. Return the sum of these numbers.
The test cases are generated so that the answer fits in a 32-bits integer.
A leaf node is a node with no children.
Example 1:
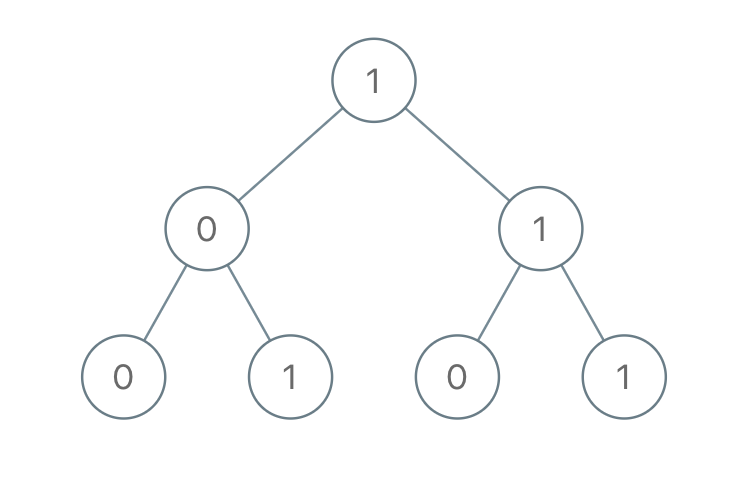
Input: root = [1,0,1,0,1,0,1]
Output: 22
Explanation: (100) + (101) + (110) + (111) = 4 + 5 + 6 + 7 = 22
Example 2:
Input: root = [0]
Output: 0
2. Sum Root To Leaf – Solution
The following is a solution which demonstrates how to get the total sum root to leaf binary numbers in a binary tree.
This solution uses Depth First Search to explore items at each level.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 |
// ============================================================================ // Author: Kenneth Perkins // Date: Jan 10, 2022 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get sum root to leaf binary numbers // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int SumRootToLeaf(TreeNode root) { return Traverse(root, 0); } private int Traverse(TreeNode node, int currentSum) { if (node == null) { return 0; } // Calculate current sum currentSum = (currentSum * 2) + node.val; // We have reached a leaf node if (node.left == null && node.right == null) { return currentSum; } // Keep traversing left and right calculating the sum return Traverse(node.left, currentSum) + Traverse(node.right, currentSum); } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
22
0
C# || How To Construct Binary Tree From Preorder And Inorder Traversal Using C#

The following is a module with functions which demonstrates how to construct a binary tree from pre order and in order traversal using C#.
1. Build Tree – Problem Statement
Given two integer arrays preorder and inorder where preorder is the preorder traversal of a binary tree and inorder is the inorder traversal of the same tree, construct and return the binary tree.
Example 1:
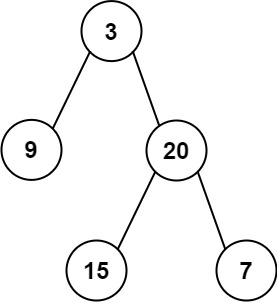
Input: preorder = [3,9,20,15,7], inorder = [9,3,15,20,7]
Output: [3,9,20,null,null,15,7]
Example 2:
Input: preorder = [-1], inorder = [-1]
Output: [-1]
2. Build Tree – Solution
The following is a solution which demonstrates how to construct a binary tree from pre order and in order traversal.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 20, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to build binary tree from pre order and in order // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { private int preorderIndex; private Dictionary<int, int> inorderIndexMap; public TreeNode BuildTree(int[] preorder, int[] inorder) { preorderIndex = 0; // Build a hashmap to store value -> its index relations inorderIndexMap = new Dictionary<int, int>(); for (int index = 0; index < inorder.Length; ++index) { inorderIndexMap[inorder[index]] = index; } return ArrayToTree(preorder, 0, preorder.Length - 1); } private TreeNode ArrayToTree(int[] preorder, int start, int end) { // If there are no elements to construct the tree if (start > end) { return null; } // Select the preorder_index element as the root and increment it var rootValue = preorder[preorderIndex++]; var root = new TreeNode(rootValue); // Get current positon var curPos = inorderIndexMap[rootValue]; // Build left and right subtree // excluding inorderIndexMap[rootValue] element because it's the root root.left = ArrayToTree(preorder, start, curPos - 1); root.right = ArrayToTree(preorder, curPos + 1, end); return root; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[3,9,20,null,null,15,7]
[-1]
C# || How To Construct Binary Tree From Inorder And Postorder Traversal Using C#

The following is a module with functions which demonstrates how to construct a binary tree from in order and post order traversal using C#.
1. Build Tree – Problem Statement
Given two integer arrays inorder and postorder where inorder is the inorder traversal of a binary tree and postorder is the postorder traversal of the same tree, construct and return the binary tree.
Example 1:

Input: inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
Output: [3,9,20,null,null,15,7]
Example 2:
Input: inorder = [-1], postorder = [-1]
Output: [-1]
2. Build Tree – Solution
The following is a solution which demonstrates how to construct a binary tree from in order and post order traversal.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 20, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to build binary tree from in order and post order // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { private int postorderIndex; private Dictionary<int, int> inorderIndexMap; public TreeNode BuildTree(int[] inorder, int[] postorder) { postorderIndex = postorder.Length - 1; // Build a map to store value & its index relation inorderIndexMap = new Dictionary<int, int>(); for (int index = 0; index < inorder.Length; ++index) { inorderIndexMap[inorder[index]] = index; } return ArrayToTree(postorder, 0, postorder.Length - 1); } private TreeNode ArrayToTree(int[] postorder, int start, int end) { // If there are no elements to construct the tree if (start > end) { return null; } // Select the postorder element as the root and decrement it var rootValue = postorder[postorderIndex--]; var root = new TreeNode(rootValue); // Get current positon var curPos = inorderIndexMap[rootValue]; // Build left and right subtree // excluding inorderIndexMap[rootValue] element because it's the root root.right = ArrayToTree(postorder, curPos + 1, end); root.left = ArrayToTree(postorder, start, curPos - 1); return root; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[3,9,20,null,null,15,7]
[-1]
C# || How To Get Total Sum Of Left Leaves In Binary Tree Using C#

The following is a module with functions which demonstrates how to get the total sum of left leaves in a binary tree using C#.
1. Sum Of Left Leaves – Problem Statement
Given the root of a binary tree, return the sum of all left leaves.
A leaf node is a node with no children.
Example 1:
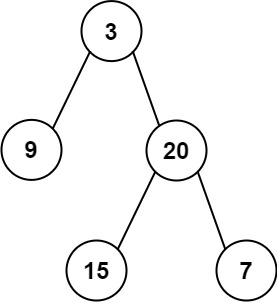
Input: root = [3,9,20,null,null,15,7]
Output: 24
Explanation: There are two left leaves in the binary tree, with values 9 and 15 respectively.
Example 2:
Input: root = [1]
Output: 0
2. Sum Of Left Leaves – Solution
The following are two solutions which demonstrates how to get the total sum of left leaves in a binary tree.
Both solutions use Depth First Search to explore items at each level.
In both solutions, we traverse the left and right side of the tree, keeping track of which node is the ‘left’ node.
When a leaf is encountered and its a node on the left side, we increment the final result with the value of the node on the left side.
Recursive
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 3, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get the sum of left leaves // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int SumOfLeftLeaves(TreeNode root) { return Traverse(root, false); } private int Traverse(TreeNode node, bool isLeft) { if (node == null) { return 0; } // We have reached a leaf node if (node.left == null && node.right == null) { return isLeft ? node.val : 0; } // Keep traversing left and right looking for left leaves return Traverse(node.left, true) + Traverse(node.right, false); } }// http://programmingnotes.org/ |
Iterative
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 3, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get the sum of left leaves // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int SumOfLeftLeaves(TreeNode root) { if (root == null) { return 0; } // Declare stack var stack = new Stack<KeyValuePair<TreeNode, bool>>(); var result = 0; // Add root to stack stack.Push(new KeyValuePair<TreeNode, bool>(root, false)); // Loop through items on the stack while (stack.Count > 0) { // Get current node and value indicating if its a left node var current = stack.Pop(); var node = current.Key; var isLeft = current.Value; // We have reached a leaf node if (node.left == null && node.right == null) { result += isLeft ? node.val : 0; } else { // Keep traversing left and right looking for left leaves if (node.left != null) { stack.Push(new KeyValuePair<TreeNode, bool>(node.left, true)); } if (node.right != null) { stack.Push(new KeyValuePair<TreeNode, bool>(node.right, false)); } } } return result; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
24
0
C# || How To Get Total Sum Root To Leaf Numbers In Binary Tree Using C#

The following is a module with functions which demonstrates how to get the total sum root to leaf numbers in a binary tree using C#.
1. Sum Numbers – Problem Statement
You are given the root of a binary tree containing digits from 0 to 9 only.
Each root-to-leaf path in the tree represents a number.
- For example, the root-to-leaf path 1 -> 2 -> 3 represents the number 123.
Return the total sum of all root-to-leaf numbers. Test cases are generated so that the answer will fit in a 32-bit integer.
A leaf node is a node with no children.
Example 1:
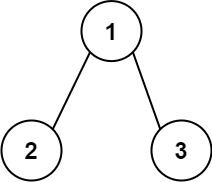
Input: root = [1,2,3]
Output: 25
Explanation:
The root-to-leaf path 1->2 represents the number 12.
The root-to-leaf path 1->3 represents the number 13.
Therefore, sum = 12 + 13 = 25.
Example 2:
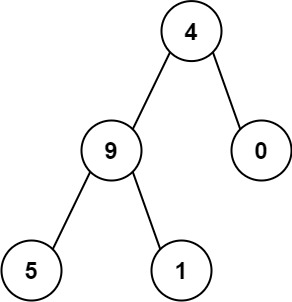
Input: root = [4,9,0,5,1]
Output: 1026
Explanation:
The root-to-leaf path 4->9->5 represents the number 495.
The root-to-leaf path 4->9->1 represents the number 491.
The root-to-leaf path 4->0 represents the number 40.
Therefore, sum = 495 + 491 + 40 = 1026.
2. Sum Numbers – Solution
The following are two solutions which demonstrates how to get the total sum root to leaf numbers in a binary tree.
Both solutions use Depth First Search to explore items at each level.
Recursive
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 3, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get sum root to leaf numbers // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int SumNumbers(TreeNode root) { return Traverse(root, 0); } private int Traverse(TreeNode node, int currentSum) { if (node == null) { return 0; } // Calculate current sum currentSum = (currentSum * 10) + node.val; // We have reached a leaf node if (node.left == null && node.right == null) { return currentSum; } // Keep traversing left and right calculating the sum return Traverse(node.left, currentSum) + Traverse(node.right, currentSum); } }// http://programmingnotes.org/ |
Iterative
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 |
// ============================================================================ // Author: Kenneth Perkins // Date: Nov 3, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Demonstrates how to get sum root to leaf numbers // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public int SumNumbers(TreeNode root) { if (root == null) { return 0; } // Declare stack var stack = new Stack<KeyValuePair<TreeNode, int>>(); var result = 0; // Add root to stack stack.Push(new KeyValuePair<TreeNode, int>(root, 0)); // Loop through items on the stack while (stack.Count > 0) { // Get current node and running sum var current = stack.Pop(); var node = current.Key; var currentSum = current.Value; // Calculate current sum currentSum = (currentSum * 10) + node.val; // We have reached a leaf node if (node.left == null && node.right == null) { result += currentSum; } else { // Keep traversing left and right calculating the sum if (node.left != null) { stack.Push(new KeyValuePair<TreeNode, int>(node.left, currentSum)); } if (node.right != null) { stack.Push(new KeyValuePair<TreeNode, int>(node.right, currentSum)); } } } return result; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
25
1026
C# || How To Traverse Binary Tree Level Order Using C#

The following is a module with functions which demonstrates how to traverse binary tree level order using C#.
1. Level Order – Problem Statement
Given the root of a binary tree, return the level order traversal of its nodes’ values. (i.e., from left to right, level by level).
Example 1:
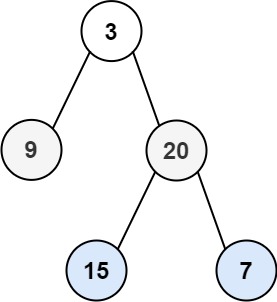
Input: root = [3,9,20,null,null,15,7]
Output: [[3],[9,20],[15,7]]
Example 2:
Input: root = [1]
Output: [[1]]
Example 3:
Input: root = []
Output: []
2. Level Order – Solution
The following is a solution which demonstrates how to traverse binary tree level order.
This solution uses Breadth First Search to explore items at each level.
|
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 |
// ============================================================================ // Author: Kenneth Perkins // Date: Oct 29, 2021 // Taken From: http://programmingnotes.org/ // File: Solution.cs // Description: Determines how to traverse a tree level order // ============================================================================ /** * Definition for a binary tree node. * public class TreeNode { * public int val; * public TreeNode left; * public TreeNode right; * public TreeNode(int val=0, TreeNode left=null, TreeNode right=null) { * this.val = val; * this.left = left; * this.right = right; * } * } */ public class Solution { public IList<IList<int>> LevelOrder(TreeNode root) { var result = new List<IList<int>>(); var queue = new Queue<TreeNode>(); if (root != null) { queue.Enqueue(root); } while (queue.Count > 0) { var level = new List<int>(); // Loop through items in the queue for (var itemCount = queue.Count; itemCount > 0; --itemCount) { var current = queue.Peek(); queue.Dequeue(); // Add children to the queue if they exist if (current.left != null) { queue.Enqueue(current.left); } if (current.right != null) { queue.Enqueue(current.right); } // Add current value to the level level.Add(current.val); } // Add items on this level to the result result.Add(level); } return result; } }// http://programmingnotes.org/ |
QUICK NOTES:
The highlighted lines are sections of interest to look out for.
The code is heavily commented, so no further insight is necessary. If you have any questions, feel free to leave a comment below.
Once compiled, you should get this as your output for the example cases:
[[3],[9,20],[15,7]]
[[1]]
[]












